Infrared Spectroscopy

To read more about my tutoring services click tutoring Mississauga

Abstract

Infrared radiation is a form of electromagnetic radiation. This region of the electromagnetic spectrum has a wavelength longer than that of visible light. Further, spectroscopy is defined as the study of the interaction between radiation and matter (in this experiment the matter been molecules of gas and some solids). Now, infrared spectroscopy is the study of how molecules interact to radiation that originates from the infrared region of the electromagnetic spectrum. The wavelength of infrared radiation is such that it entices the vibrational modes of the sample in question.

Introduction

TThe internal energy of a molecule is associated with the atomic bonds of the molecule. This type of energy is broken down into three components such as electronic, vibrational and rotational. Electronic transitions are transitions where the valence electron of the molecule is excited to a higher energy level. These types of transitions usually occur in the ultraviolet and visible regions of the electromagnetic spectrum. Moreover, rotational transitions are transitions where the angular momentum of the molecule is changed. These transitions occur when microwave radiation is bombarded on the molecule. Further, there are different types of vibrations and these include vibrations of an oscillatory electric dipole change and the absorption or emission of electromagnetic radiation. This occurs in the mid-infrared region where most molecules tend to have their natural frequencies. Moreover, in this experiment the vibrational absorption spectra of samples in solid and gaseous states will be observed and analyzed.

Theory

The infrared region of the electromagnetic spectrum has three components to it. These components include the near-infrared, mid-infrared and far-infrared regions and the manner is which they are named is in relation to the visible spectrum. The near-infrared region which has the highest energy of these components has the range of wave numbers from 14000 cm-1 to -4000 cm-1. The mid-infrared region which is used in the study of rotational-vibrational fine structure of the molecules has a range of wave numbers from 4000 cm-1 to 400 cm-1. Further, the far-infrared region has the lowest energy and is used in the study mainly of the rotational structure of the molecules and this region has a range of wave numbers from 400 cm-1 to 10 cm-1.

Molecules have certain frequencies at which they can vibrate or rotate. These frequencies exploit the fact that these molecules would rotate or vibrate only to corresponding energy levels or vibrational modes. Moreover, for a vibrational mode to respond to infrared radiation the molecule has to be associated with a permanent dipole. The manner in which an infrared spectrum that can be obtained from a sample is by bombarding the sample with infrared radiation. In this experiment the instrument that is used to produce the mid-infrared region of the spectrum is the Perkin-Elmer 983G Spectrophotometer. This instrument has a range of 5000 cm-1 to 180 cm-1. The machine basically is a microprocessor controlled double-beam dispersion device. It has a heated ceramic source with a thermocouple detector and four diffraction gratings which correspond to different frequency settings. A diffraction grating is rotated for the purpose of the radiation of different frequencies to reach the thermocouple detector while the device is set to scan.

Furthermore, the Beer-Lambert Law is a relationship between the absorption of light and the properties of the material in which the light is absorbed into. The amount of intensity of light transmitted through a sample of thickness, x, is give by:

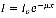1

Where 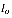term is the incident intensity of the beam and μ is the absorption coefficient which is a function of frequency. Hence, the transmittance is defined in the following manner:

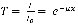2

Here the plot consists of a %T in the double beam mode. Moreover, the absorbance is defined as:

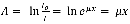3

It can be seen from equation (3) that the absorption is proportional to 5 where 6 is the thickness.

Moreover, for the vibrational and rotational bands for Carbon Monoxide gas there is only one vibration which is the C-O stretch. Once a spectrum is obtained of these molecules various quantities can be determined such as the force constant between the C-O bond (K), the average bond length of the vibrating molecule (ro) and finally the relative populations of various rotational levels (Nj).

The bond between C-O can be modelled as a harmonic oscillator. Hence, the vibrational energy can be represented by:

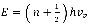4 where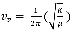

where n represents the vibrational quantum number and can takes on integer values, 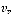is defined as the fundamental vibrational frequency and 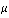is the reduced mass of the system and finally K represents the force constant. Further, each vibrational level has a rotational fine structure. The energy for a given vibrational level is represented by:

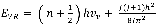5

where J is rotational quantum number which takes on integer values and is the moment of inertia about an axis through the center of mass. In this experiment, the absorption will be documented with energy levels ranging from 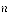= 0 to 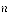= 1 for values of 1 (R branch) and -1 (P branch). For the C-O molecules the Q branch corresponds to the = 0 which is not infrared active. For 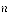= 0 the R branch is = 0 and the P branch is = 1. These lines can be represented by:

R ( ) = R(0) = σv + 2B( + 1) = σv + 2B (6)
and
P ( ) = P(1) = σv - 2B( ) = σv - 2B (7)

where B = 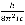and c represents the speed of light. Once B is determined the value for can be determined via the formula:

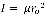8

The average bond length of the vibrating molecule, ro, can be determined from this data. Moreover, the manner in which the relative populations of various rotational levels, Nj, are determined is by the Boltzmann distribution:

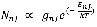9

where 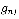is defined as the statistical weight. In this experiment, the absorption bands are in the ground vibrational state (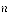) = 0 ) so:

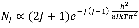10

Further, if equation (10) is differentiated with respect to 1 and then set to zero a maximum value for 1 can be obtained:

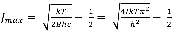11

Procedure

The procedure that was followed was outlined in the write-up provided for Experiment #10.

Discussion

Part I: Atmospheric Absorption
For the single beam the most prevalent dip within the graph was observed to be at 2366.5 cm-1. For the double beam the most prevalent dip that was observed occurred at 2322.3 cm-1. This number is well within the range for the absorption of CO2 and the mode is exemplifies is the asymmetric stretch.

Part II: Beer-Lambert Law of Absorption The Beer-Lambert law of absorption was put to the test by the use of various Mylar thicknesses. To be exact, six Mylar thicknesses were used. The absorbance of this material proved to be charismatic in the output of the graph. Moreover, the absorption coefficient, 1, was determined to be 65.7 cm-1.

Mylar Thickness (µm)	% Absorbance
38.1	0.5833
31.7	0.5296
25.4	0.4277
19.0	0.3383
12.7	0.2279
6.3	0.1138

Part III: Vibrational-Rotation Bands for Carbon Monoxide Gas

Force Constant (K)

In order to calculate the force constant between C-O the following equation was used:



The reduced mass was calculated to be 1.14×10‾²³kg. The fundamental vibrational frequency was determined to be 6.5×10¹³ Hz. From this information the force constant was determined to be 1.90 x 106 N/m. This is a relatively high force constant for the C-O stretch.

Average bond length of vibrating molecule

Moreover, for the calculation of the average bond length the spacing’s between the peaks were determined. This spacing was calculated to be 3.8 cm. Further, B was calculated to be 2.02 cm-1. From this value, 1, was determined to be 1.38 x 10-44 m2kg. Now the equation' 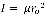, can be used to determine the bond length of C-O. This value was calculated to be 3.48 x 10-11 m.

Conclusion

In conclusion, it was determined that the double beam of the system proved to be a more reliable source for determining the mode of the CO2. The double beam mainly gave a much better graph and a better picture of the light that was transmitted. The absorption coefficient, 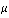, was determined to be 65.7 cm-1. Further, the force constant was determined to be 1.90 x 106 N/m, and the bond length for C-O was also calculated to be 3.48 x 10-11 m.

References

“E10 Infrared Spectroscopy”, University of Waterloo, Physics 360A, 2008.